How Does Knowing the Double 6+6=12 Help You Solve

March 12, 2022 Post a Comment

Basic Facts Lesson 3: Knowing and using doubles to add

Derived facts.

Using derived fact strategies comes later learning counting strategies to solve addition and subtraction problems. A derived fact strategy means that there are some number combinations that the child knows and has memorized, and he or she uses those combinations to figure out (derive) other number combinations.

Children oftentimes memorize double facts fairly early on on, so learning how to use doubles to figure out near-double facts is ane of the outset derived fact strategies children are able to use.

Doubles

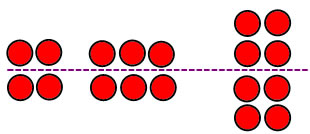
Before children can use doubles to solve other problems, they must beginning learn some of the doubles. Edifice pictures for doubles by using symmetry is 1 way for children to learn doubles, peculiarly for doubles of numbers to v. For example, children can place counters on either side of a symmetry line to evidence the double facts:

counters showing doubles

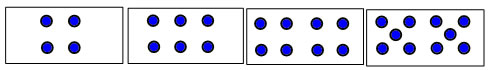
The standard dot cards suggested in the previous lesson likewise have dots grouped to show doubles:

dot cards showing doubles

As suggested in the Teaching Student-Centered Mathematics text, you can besides make pictures or wink cards with common objects that show doubles. Unfortunately, it'southward rather difficult to find good examples for some of the larger doubles:

pictures showing doubles

Following the philosophy that children should always have a thinking strategy for figuring things out before they undertake practice and memorization tasks, it's worth noting a couple of thinking strategies for figuring out the larger doubles (doubles higher up v+5).

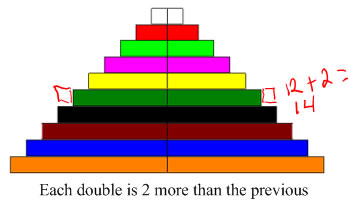
I strategy is well illustrated by a double Cuisenaire rod staircase. Information technology shows how each double is ii more than the last double, so if you know 6+six=12, and then you tin can figure out vii+seven=12+two=14

Cuisenaire rod double stair case

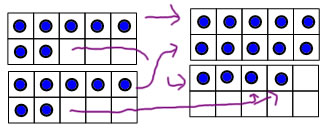
Another strategy is a 10-frame strategy that is sometimes called the "ii 5's" strategy: if you lot can visualize 7 as 5+ii, then y'all tin can see that the ii rows of v make 10, and the ii two's make 4 more then 7+7=14:

two fives

Of course, in one case children have skillful ways of figuring out double facts, and so it'southward time to practice so that at least some of them are memorized. Practice tin can exist just flash cards, or it can exist in the grade of songs or rhymes or games. A lot of games can exist modified to practice double facts--just provide i 10-sided die, and make the rule that y'all double the number on the die and become forward that many spaces, or collect that many points or tokens.

Using doubles

An introductory lesson using a word problem... Permit'southward call up about a grade where the children take talked about and adept doubles a little fleck each day for several days (perhaps 2-3 weeks) and all of the children know at to the lowest degree some of the double facts. The teacher wants to introduce the idea of using double facts to solve facts that are virtually doubles. She might ask the class to recollect most the trouble:

Sarah has 6 red balloons and 7 blue balloons. How many balloons does Sarah accept?

Later waiting for children to call up and figure out a solution, she asks: who would like to share how they solved this problem?

Allow's look behind the scenes: what did the teacher do when she prepared the question? First, she chose a PPW-WU problem--she wants to showtime out with a problem that doesn't have a change over time, considering JRU change over fourth dimension issues suggest counting on quite strongly, and she is hoping children volition utilize the doubles they have been practicing instead of counting on. 2nd she chose numbers that are large enough that counting on isn't necessarily the obvious thing to do, and she chose numbers that are only one abroad from the double half dozen+6. The double of six is ordinarily learned before the doubles of vii, 8 and 9, so she'due south giving a problem that is close to a double well-nigh children will know confidently. At present, she is hoping that some children will have used a double fact to effigy out the trouble.

Lets wait at the scenarios that might play out next:

What if... Children solved the trouble in several different means. Someone volunteers a counting on strategy, someone else describes using 6+vi and adding one more, and nonetheless another kid describes using seven+vii and taking one away.

This is just what the teacher is hoping for. Afterward listening to several strategies, she reminds the children of the two strategies that used doubles. She describes those strategies again, and and then invites the children to endeavor solving the adjacent problem by thinking well-nigh a double that tin assist them. She then poses a similar problem:

Michael has viii crayons.
Kyle has 7 more crayons than Michael.
How many crayons does Kyle take?

She has inverse the problem type to some other addition problem type, now that she is specifically asking children to think about doubles, and she has inverse the numbers, but they are all the same just one more than (or one less) than a double.

Later children take had a few minutes to call up, she follows up with some double questions:

Who can tell me a double that might help us to solve this problem?
Who used that double?
How did y'all use the double to notice the answer to this problem?
Did anyone use a different double to help them?
How did you use that double to solve the problem?

And in that location you have a sample introductory mini-lesson for introducing the strategy of using doubles to add together. Lets think for a minute near what variations at that place might be on a lesson similar this:

What if... No one volunteered a strategy that used doubles? What could the teacher practise and then...

She could utilise questions to assistance children recollect about using doubles:

I was wondering if we could use any of the doubles nosotros've learned to help us solve this problem...
Tin anyone think of a double that would be close to 6+7?
Could we employ six+6 to figure out 6+7?

She could model the thinking she wants children to do:

I in one case saw someone solve the problem this way...mind and see if you can effigy this out... If she had half-dozen cherry and half-dozen blueish balloons that would be 12 because double half-dozen is 12. six cherry-red and 7 blueish balloons would be merely i more than, so that would exist xiii balloons.

In both cases she would follow up with another problem. Probably she would want to make the follow upward question more than similar to the first trouble she gave considering she would take lesson indication that the children were really set up and confident. She might choose a problem similar:

Mandy has 5 Silly Bandz. Cheryl has half-dozen Silly Bandz. How many Silly Bands do they have all together?

Again, she would follow up with the same sorts of questions that are focused on using doubles:

Who can tell me a double that might help us to solve this problem?
Who used that double?
How did you use the double to find the respond to this problem?

Lesson variations:

Likewise math talks nigh word problems with addends that are virtually doubles, some other good lessons and discussions are:

Showing near double problems on a pair of 10-frames is a good mode to illustrate and hash out strategies.
Choose a near doubles number problem such equally vii+8 and enquire what doubles could yous use to figure out this trouble?
Choose several number problems, some with near doubles, and some where the numbers are further apart, and ask: which of these can we apply doubles to help united states of america solve? Which ones are easiest to solve using doubles facts?
Games make good practice. This site has a expert game for practicing near doubles.

Things to know about writing problems for using doubles:

As a teacher, yous are selecting appropriate bug for children to solve--ones that are appropriate and challenging for their cognition, but non and so hard that they have to revert to more concrete modeling solutions. When children are learning how to use doubles to figure out other issues, they need to spend time at commencement working just with problems where using doubles is an appropriate and efficient matter to do.

Doubles +one or -one: The easiest issues to apply knowledge of doubles to are the bug that are just one away from being doubles: doubles +one and doubles -i:

3+iv
four+5
v+6
half-dozen+7
7+8
eight+ix

iv+3
5+4
6+5
7+6
8+seven
9+8

It isn't that the ones on the left are doubles +1 and the ones on the correct are doubles -one, its that children will choose to use a doubles +1 or -ane strategy depending on what doubles they know best. So, for instance, 7+8 tin be solved from seven+7 with a doubles +1 strategy, and can be solved from viii+viii with a doubles -i strategy. Then, depending on whether the child recognizes and knows 7+7 or eight+8 with more confidence, they can apply a doubles +i or a doubles -1 strategy to the problem.

Issues can be solved by a doubles +ane or a doubles -1 strategy if the 2 addends are simply 1 autonomously.

Using a doubles +ane or a doubles +1 strategy requires 3 steps:

recognize that ane addend is just one greater than the other in the trouble
know the double fact for i of the addends
add or subtract ane from the appropriate double fact.

Doubles +2 or -2: The adjacent ready of problems that children can solve using doubles, are the ones that can be solved with a doubles +2, a doubles -two or a sandwich doubles strategy. Bug can be solved past a doubles +2 or a doubles -2 strategy if the difference betwixt the addends is 2 (ane of them is 2 more than or ii less than the other).

3+five
4+6
5+7
half dozen+viii
7+nine

5+3
six+4
seven+5
8+6
nine+7

There are three strategies that can be used to solve these issues. I'll utilise 8+6 as an case:

Doubles +2: If I know half-dozen+half dozen=12, and I run into that 8 is ii more than half dozen, then I can solve 8+6 past adding 12+two=fourteen.

Doubles -2: If I know 8+8=16 and I come across that vi is 2 less than 8, and so I tin can solve viii+6 by subtracting 16-ii=14

Using a doubles +two or a doubles +2 strategy requires three steps:

recognize that 1 addend is merely two greater than the other in the trouble
know the double fact for one of the addends
add or subtract 2 from the appropriate double fact.

Sandwich doubles: If I realize that 6 is ane less than seven, and 8 is one more than 7 (7 is sandwiched in between the other two numbers), then I tin requite 1 from the 8 to the 6 to become 8+6=7+7=xiv. ten-frames are good to illustrate this strategy:

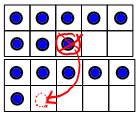
sandwich doubles pic

This strategy is a flake more than sophisticated than the other 2, and it doesn't accept a standardized proper noun (doubles +2 and doubles -2 are the names that everyone uses; sandwich doubles is a proper name I fabricated upward or I read somewhere or something), but it's adequately common as a strategy for children to invent. All of these strategies are ones where children are the best inventors, and the instructor's main task is to ask good questions.

Using the sandwich doubles strategy requires 3 steps:

recognize that one addend is one greater and one addend is one less than the same number
know the double fact for the centre number
realize that the two sums are the same because y'all can give one from the smaller addend to the lesser addend..

Word problem types: Employ doubles strategies rely on comparison the sizes of the ii addends, so it's best to start with a static problem blazon when introducing problems that utilize doubles: PPW-WU is a particularly practiced i for this. After children have some experience with using doubles to solve problems, the teacher should exist varying the problem types to include the other main addition trouble types: CQU (with a more...than structure) and JRU.

Efficiency: Problems where the addends take a difference of 1 or 2 are generally considered to be bug where using doubles is an efficient strategy to utilise. When children are practicing the utilize doubles strategy, they should spend some fourth dimension practicing just problmes where using doubles is an efficient strategy.

Timed tests: to work on speed while practicing a detail strategy (like using doubles) it's a good idea to make shorter tests that work on just the problems relevant for this strategy. These tests might have the same problems repeated several times on the same page--just in a dissimilar lodge. Rathmell's essays on bones facts drills take some good ideas for this.

Summary

Children need to know many double facts before they can commencement to use a nearly doubles strategy, merely they don't have to know all of them.
Children likewise need to be able to recognize when ane number is only i more or two more than another.
Symmetry is a good tool for illustrating double facts.
10-frames are a good tool for explaining strategies, including double facts.
10-frames are also good for explaining most double strategies.
PPW-WU problems are better for introducing near doubles than are JRU problems beacuse they don't suggest counting on as strongly.
Children can choose one of several strategies (doubles +one or -1; doubles +two or -ii or sandwich doubles) for a single improver fact depending in which doubles they know best.
Doubles +-one are generally easier than doubles +-2.
Using doubles is by and large considered an efficient strategy only if the addends accept a difference of merely 1 or 2.
Good questions to help children figure out the use doubles strategy are:
- Can you think of a double fact that is close to ____?
- Can you recall of a double fact that could help usa figure out ____?
- What doubles fact is shut to ____?
- How tin can nosotros use the double fact ____ to help usa effigy out ___?
The doubles +-1 and doubles +-2 strategies require children to know how to exercise three things (prior knowledge and skills children must have to apply these strategies):
- recognize that two addends are close to each other, and know how much more one is than the other (in the instance where ane is one more or 2 more than the other)
- know the double fact for at least one of the addend
- add together or subtract 1 or 2 from the double.